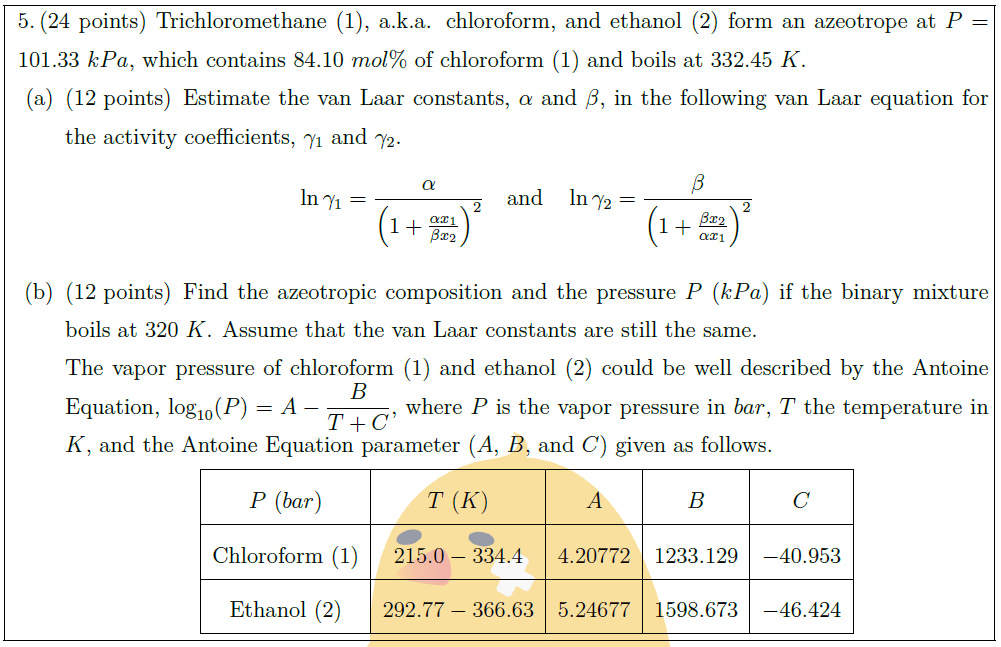
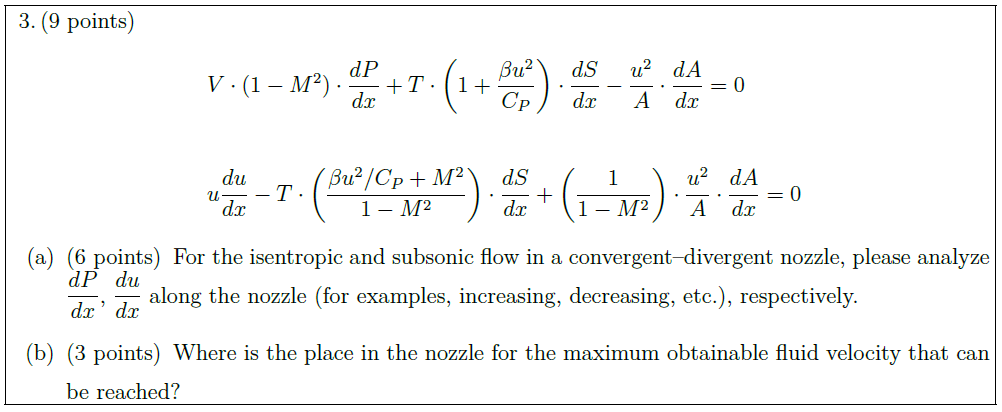
Solution:
Consider a certain gas whose $PVT$ behavior is governed by Dieterici equation of state :
\begin{align*}
P (V – b) = RT \exp \left( – \frac{a}{RTV} \right),
\end{align*}
where $a$ and $b$ are constant parameters (but not necessarily positive). Please answer the following questions :
\begin{parts}
\part [10] Suppose $\left| b/V \right| \ll 1$ and $\left| a/RTV \right| \ll 1$. Expand the above equation of state as a virial equation of state in the form : $PV/RT = 1 + B/V + C/V^2 + \cdots$. Determine the $2^{\mbox{\scriptsize nd}}$ virial coefficient, $B$, and the $3^{\mbox{\scriptsize rd}}$ virial coefficient, $C$.
\part [12] Now consider the virial equation of state $\uline{\mbox{up to the }2^{\mbox{\scriptsize nd}}\mbox{ virial term}}$ in (a). Suppose that the gas undergoes $slow$ isothermal compression. If the work needed for accomplishing this compression is always greater than that of an ideal gas, the parameters $a$ and $b$ must satisfy a certain criterion. What is the criterion? Explain your answer physically.
\part [3] Follow (b). If the compression is $suddenly$ performed, will the work here to be greater or less than that in (b)? Why?
\part [8] Suppose that the gas can be condensed into liquid at a sufficiently low temperature. This transition can only happen when $a$ and $b$ have proper signs (i.e. $> 0$ or $< 0$). What the signs of $a$ and $b$ should be? Why?
\end{parts}