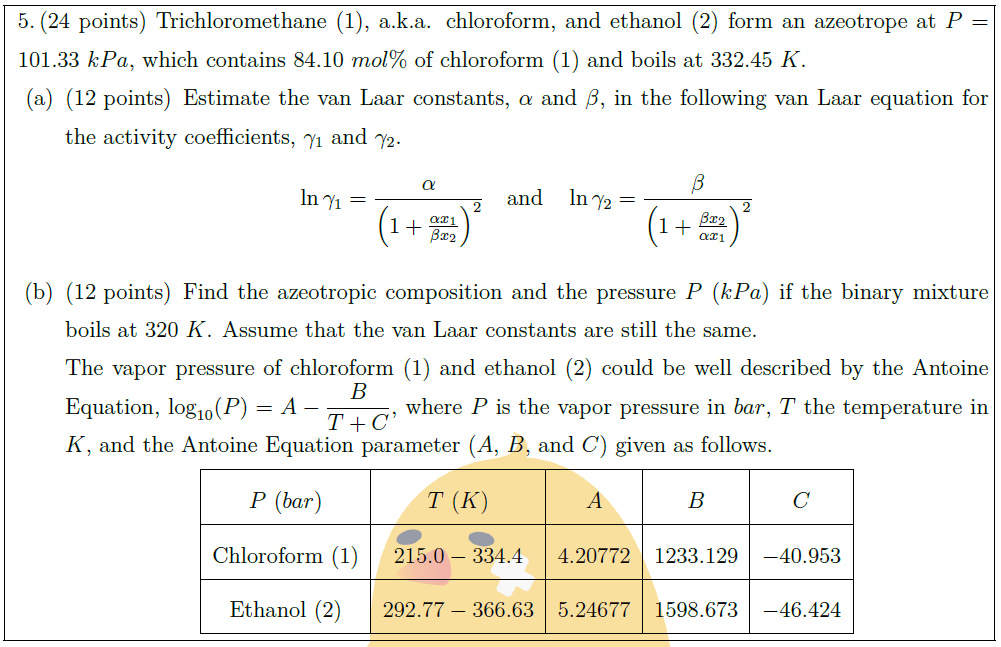
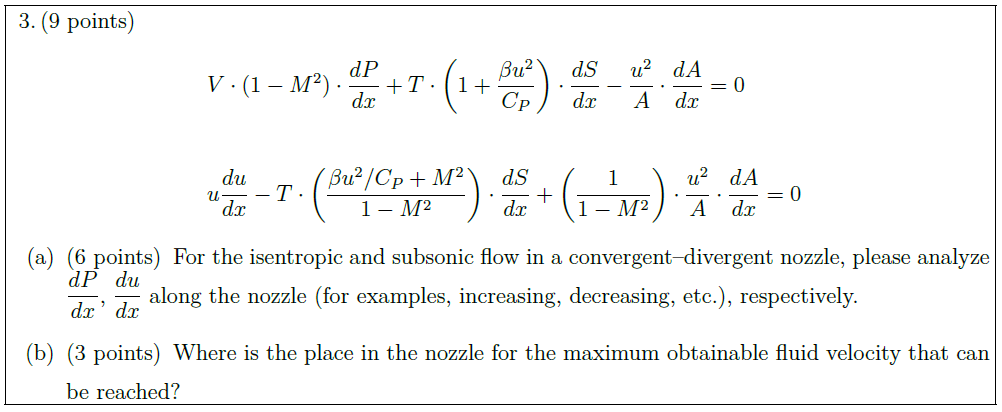
Solution:
{\bf Answer “True” or “False”. If your answer is “false”, you} $\uline{\mbox{{\bf MUST}}}$ {\bf explain it.}
\begin{parts}
\part No process is possible which consists of the transfer of heat from one temperature level to a higher one.
\part The residual property $M^R$ is defined as $M^R \equiv M – M^{ig}$, where $M$ and $M^{ig}$ are the actual and the ideal–gas values of the thermodynamic property at the same $T$ and $P$.
\part The relation $T dS + V dP = – u du$ is valid for the homogeneous phase with change between equilibrium states, which is generated from the energy balance for the adiabatic, steady–state / steady (one–dimensional; single influent / single effluent) flow of a fluid in the absence of shaft work and of changes in potential energy.
\part For one kind of the internal combustion engines, Otto cycle (Otto engine), it is also a traditional four–struck piston–cylinder process. Before combustion, the temperature of the Otto engine is extremely high as to ignite the combustion automatically.
\part Considering a heat engine as a system, then, the entropy change of the heat engine should not be less than zero.
\part $\left< C_P^{ig} \right>_S = \frac{\int_{T_0}^{T} C_P^{ig} dT / T}{(T – T_0)}$, where $\left< C_P^{ig} \right>_S$ denotes a mean value of specific heat capacity for the calculation of entropy change caused by temperature change.
\end{parts}