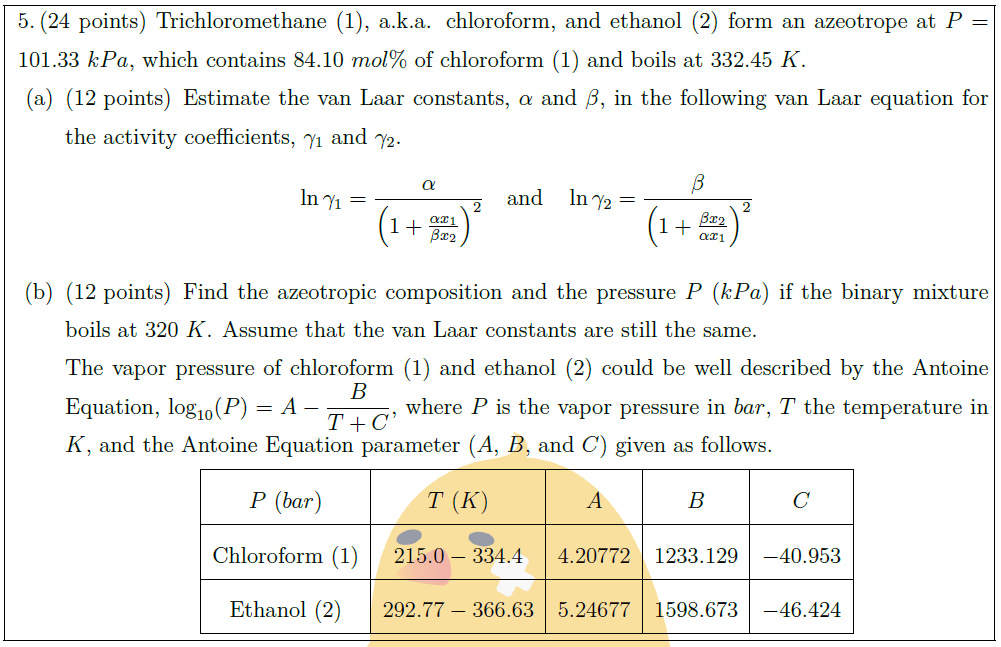
Solution:
\begin{align*}
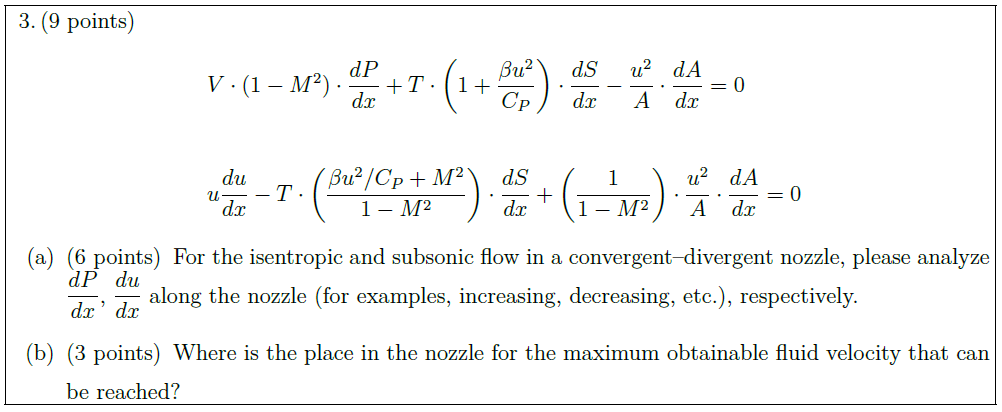
& V \cdot (1 – M^2) \cdot \frac{dP}{dx} + T \cdot \left( 1 + \frac{\beta u^2}{C_P} \right) \cdot \frac{dS}{dx} – \frac{u^2}{A} \cdot \frac{dA}{dx} = 0\\
\\
& u \frac{du}{dx} – T \cdot \left( \frac{\beta u^2 /C_P + M^2}{1 – M^2} \right) \cdot \frac{dS}{dx} + \left( \frac{1}{1 – M^2} \right) \cdot \frac{u^2}{A} \cdot \frac{dA}{dx} = 0
\end{align*}
\begin{parts}
\part [6] For the isentropic and subsonic flow in a convergent–divergent nozzle, please analyze $\displaystyle \frac{dP}{dx}$, $\displaystyle \frac{du}{dx}$ along the nozzle (for examples, increasing, decreasing, etc.), respectively.
\part [3] Where is the place in the nozzle for the maximum obtainable fluid velocity that can be reached?
\end{parts}