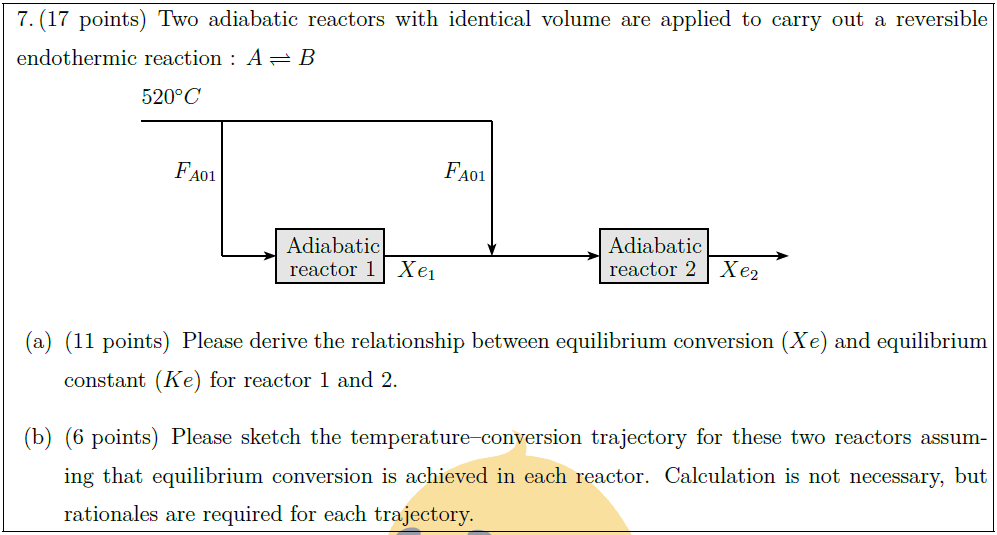
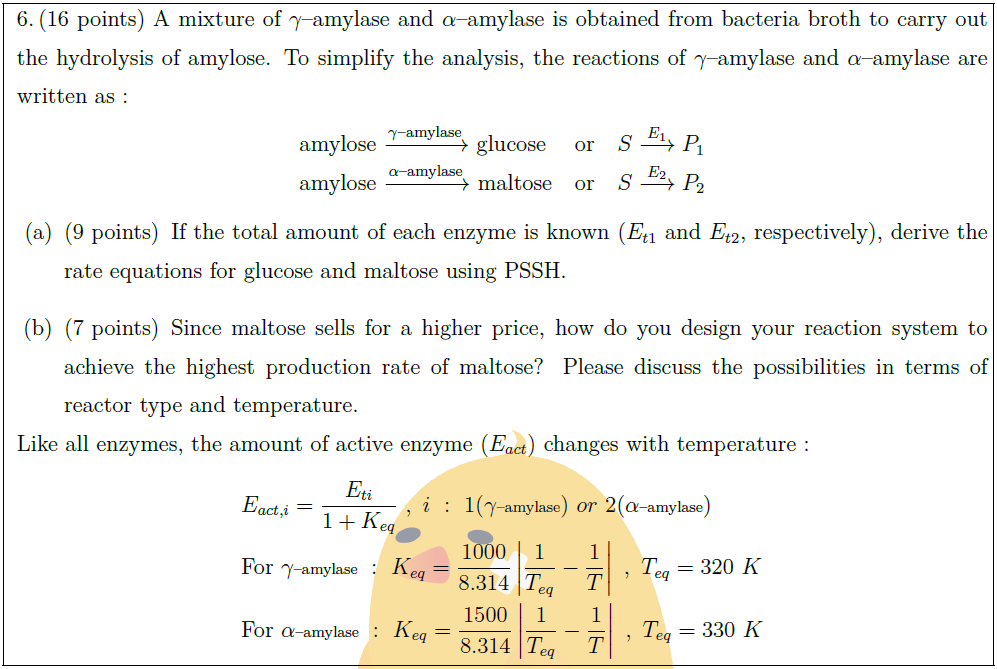
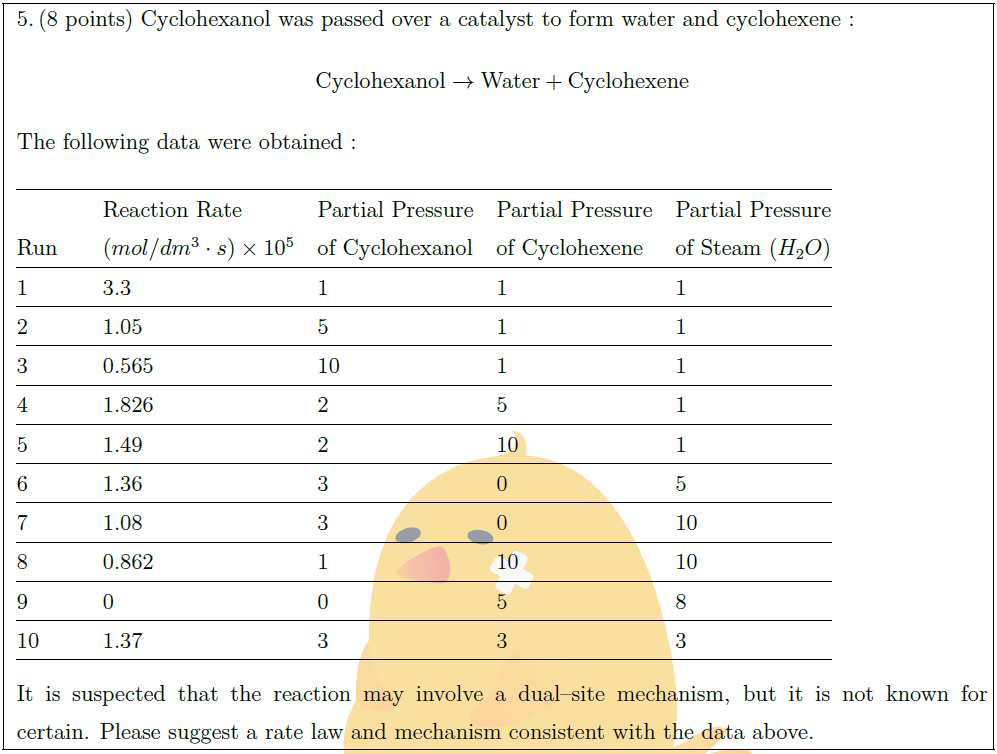
Solution:
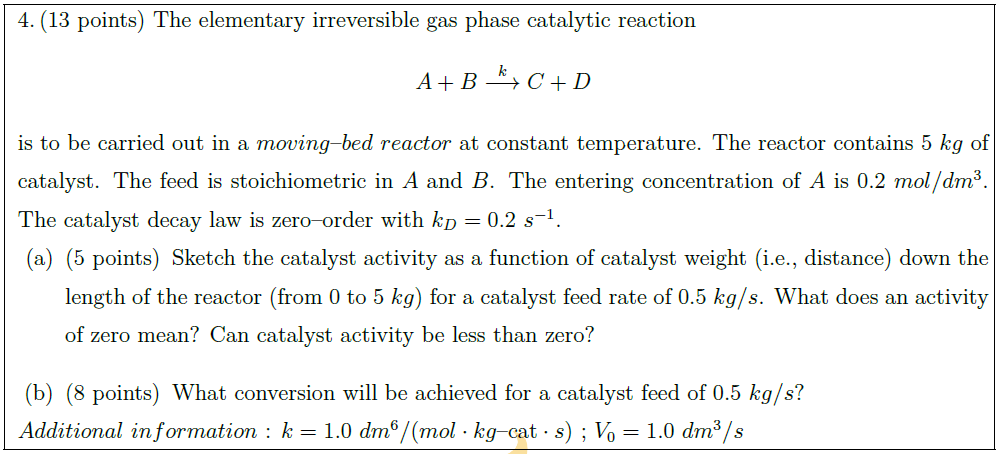
The elementary irreversible gas phase catalytic reaction
\begin{align*}
A + B \overset{k}{\longrightarrow} C + D
\end{align*}
is to be carried out in a $moving$–$bed\ reactor$ at constant temperature. The reactor contains $5\ kg$ of catalyst. The feed is stoichiometric in $A$ and $B$. The entering concentration of $A$ is $0.2\ mol/dm^3$. The catalyst decay law is zero–order with $k_D = 0.2\ s^{-1}$.
\begin{parts}
\part [5] Sketch the catalyst activity as a function of catalyst weight (i.e., distance) down the length of the reactor (from $0$ to $5\ kg$) for a catalyst feed rate of $0.5\ kg/s$. What does an activity of zero mean? Can catalyst activity be less than zero?
\part [8] What conversion will be achieved for a catalyst feed of $0.5\ kg/s$?
\end{parts}
$Additional\ information$ : $k = 1.0\ dm^6/(mol \cdot kg \mbox{–cat} \cdot s)$ ; $V_0 = 1.0\ dm^3/s$