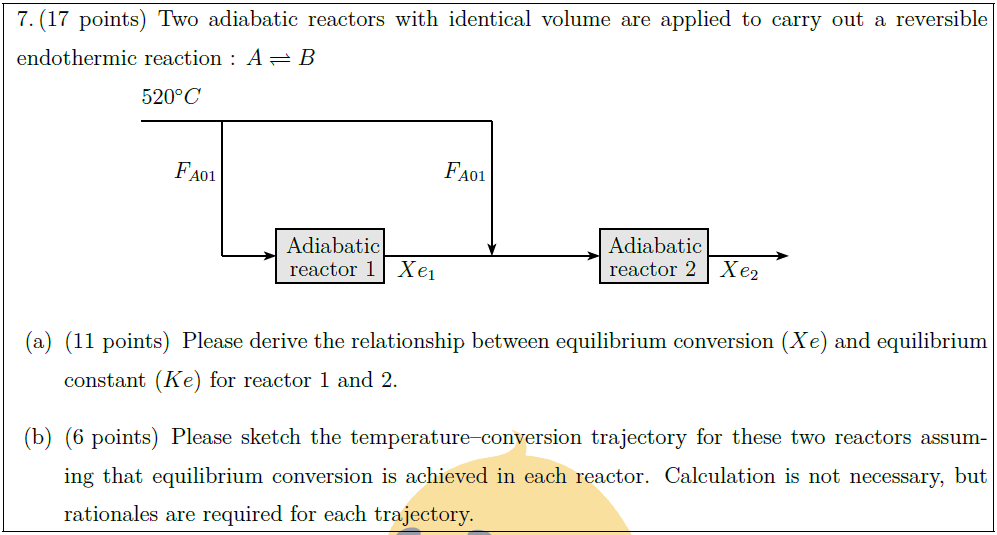
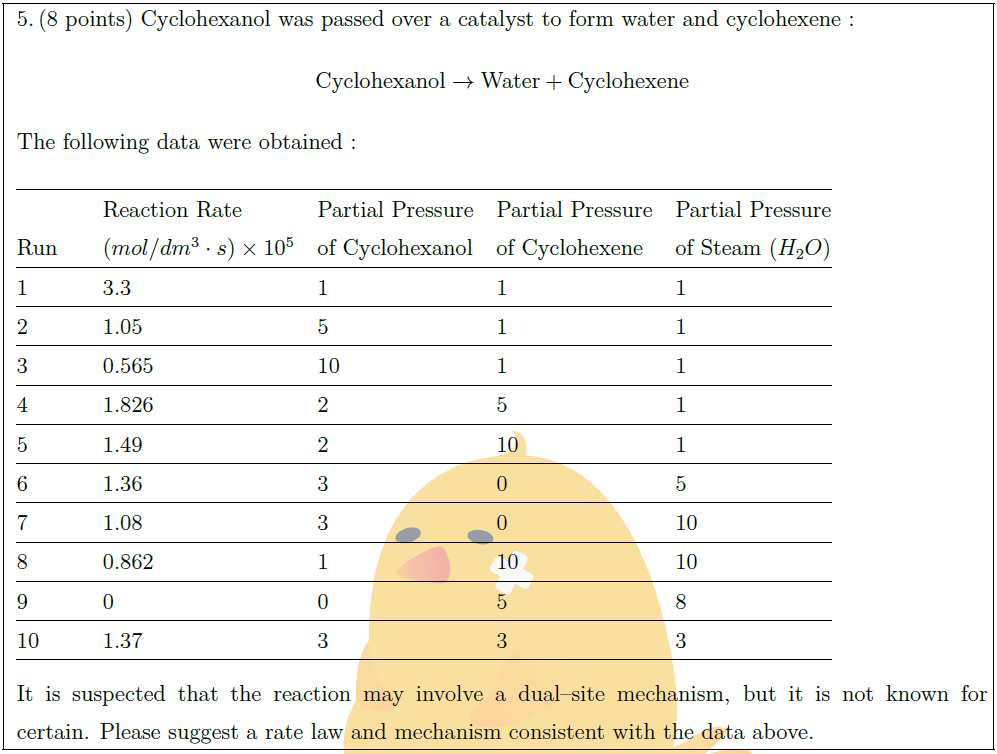
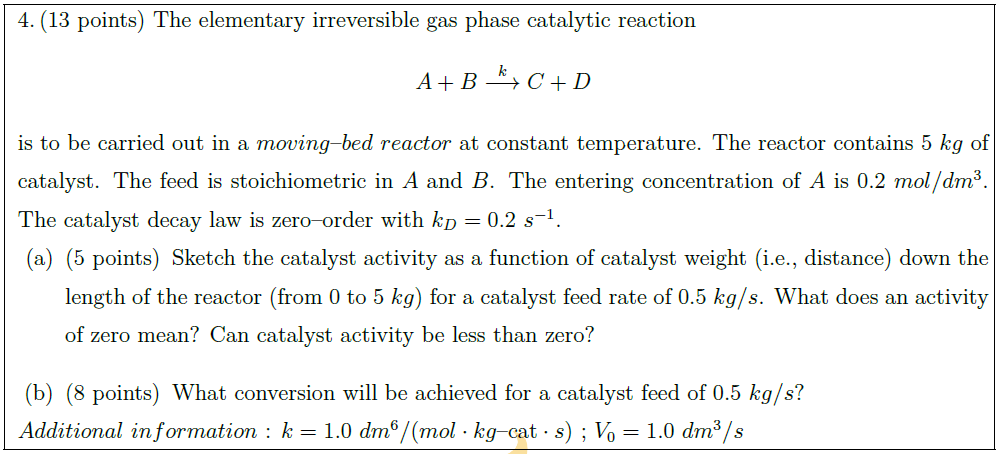
Solution:
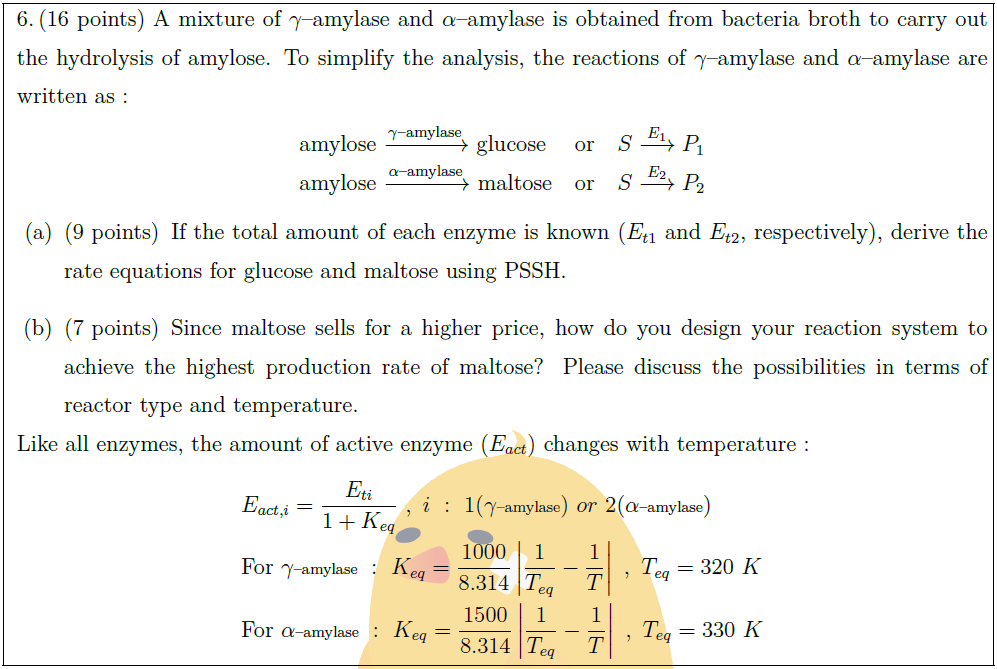
A mixture of $\gamma$–amylase and $\alpha$–amylase is obtained from bacteria broth to carry out the hydrolysis of amylose. To simplify the analysis, the reactions of $\gamma$–amylase and $\alpha$–amylase are written as :
\begin{center}
\begin{tabular}{@{}lll@{}}
amylose $\xrightarrow{\gamma \mbox{\scriptsize –amylase}}$ glucose & or & $S \overset{E_1}{\longrightarrow} P_1$\\
amylose $\xrightarrow{\alpha \mbox{\scriptsize –amylase}}$ maltose & or & $S \overset{E_2}{\longrightarrow} P_2$
\end{tabular}
\end{center}
\begin{parts}
\part [9] If the total amount of each enzyme is known ($E_{t1}$ and $E_{t2}$, respectively), derive the rate equations for glucose and maltose using PSSH.
\part [7] Since maltose sells for a higher price, how do you design your reaction system to achieve the highest production rate of maltose? Please discuss the possibilities in terms of reactor type and temperature.
\end{parts}
Like all enzymes, the amount of active enzyme ($E_{act}$) changes with temperature :
\begin{align*}
& E_{act, i} = \frac{E_{ti}}{1 + K_{eq}}\ ,\ i\ :\ 1(\gamma \mbox{\scriptsize –amylase})\ or\ 2(\alpha \mbox{\scriptsize –amylase})\\
& \mbox{For }\gamma \mbox{\scriptsize –amylase}\ :\ K_{eq} = \frac{1000}{8.314} \left| \frac{1}{T_{eq}} – \frac{1}{T} \right|\ ,\ T_{eq} = 320\ K\\
& \mbox{For }\alpha \mbox{\scriptsize –amylase}\ :\ K_{eq} = \frac{1500}{8.314} \left| \frac{1}{T_{eq}} – \frac{1}{T} \right|\ ,\ T_{eq} = 330\ K
\end{align*}