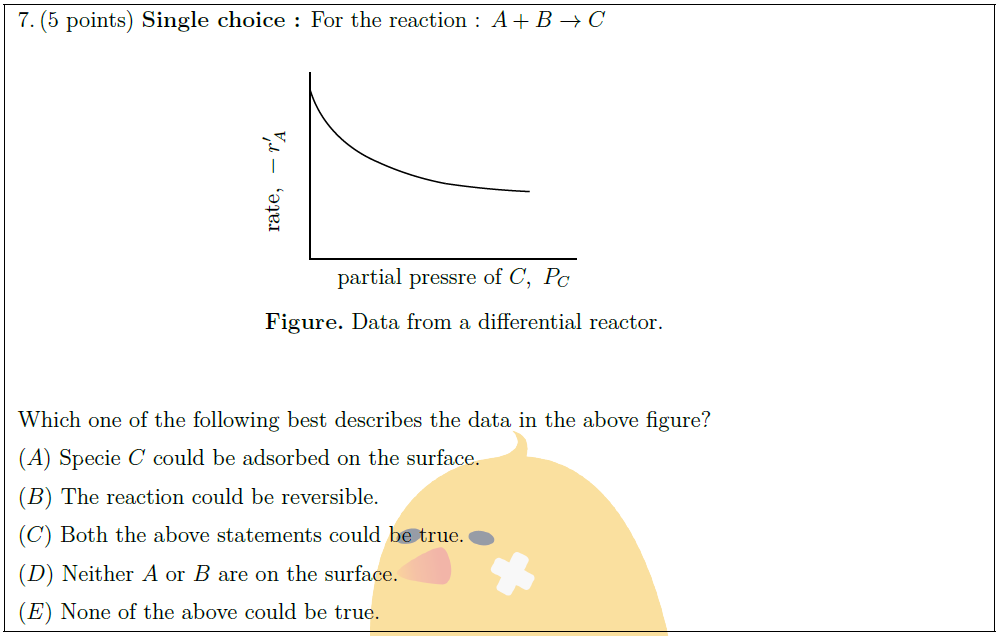
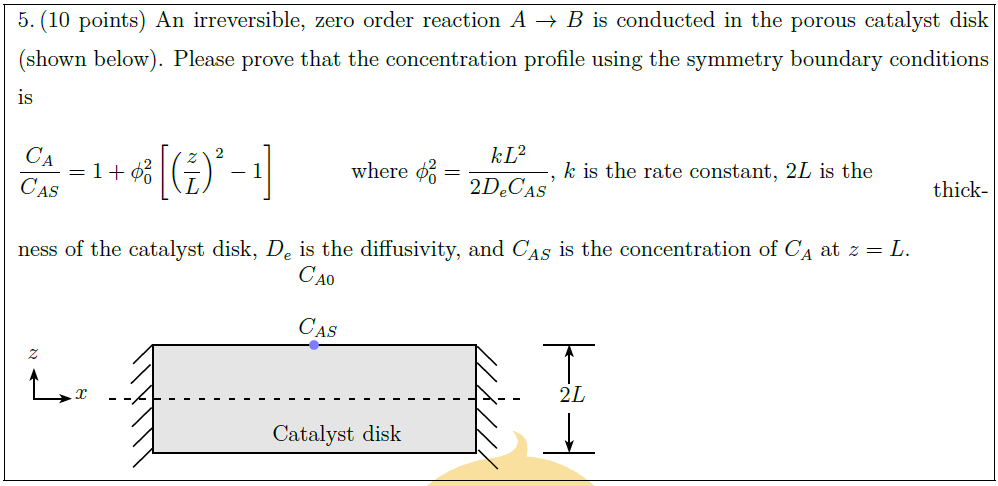
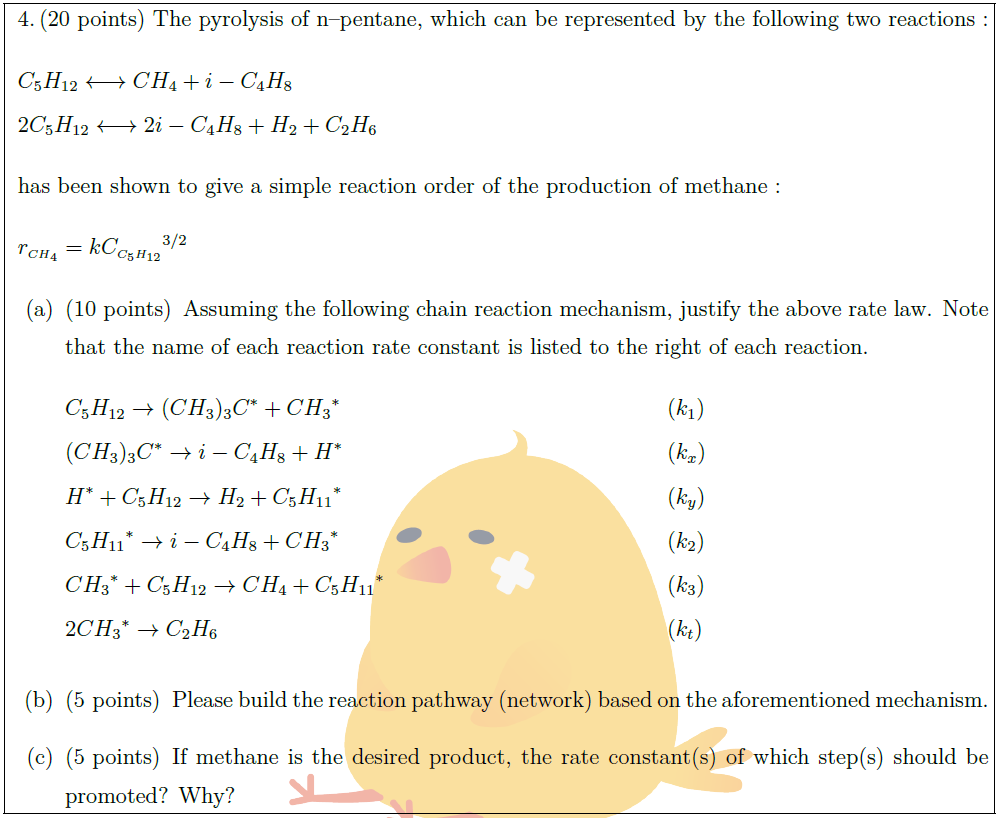
Solution:
The elementary irreversible gas–phase catalytic reaction
\begin{flalign*}
& A \overset{k_1}{\longrightarrow} B &
\end{flalign*}
is carried out isothermally in a batch reactor. The catalyst deactivation follows a first–order decay law and is independent of the concentrations of both $A$ and $B$.
\begin{parts}
\part[5] Make a qualitative sketch of catalyst activity as a function of time. Does $a(t)$ ever equal zero for a first–order decay law?
\part[10] Calculate the conversion in the reactor after 10 min–uses.
\end{parts}
Additional information :\\
$C_{A0} = 1\ mol/dm^3$ ; $V_0 = 1\ dm^3$ ; $W = 1\ kg$ ; $k_d = 0.1\ min^{-1}$ ; $k_1 = 0.2\ dm^3/ (kg\ \mbox{cat} \cdot min)$