Solution:
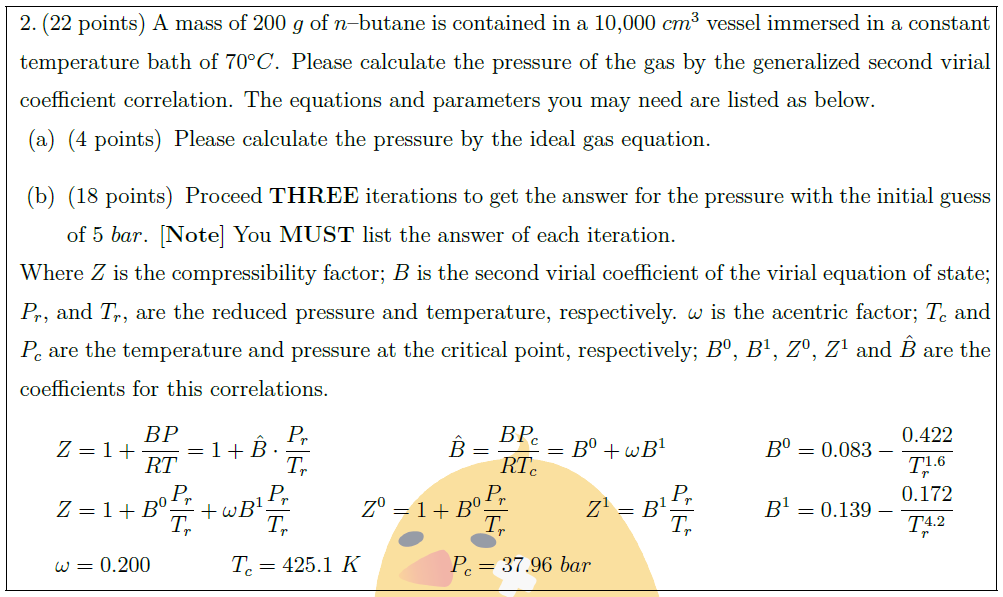
A mass of $200\ g$ of $n$–butane is contained in a 10,000 $cm^3$ vessel immersed in a constant temperature bath of $70^\circ C$. Please calculate the pressure of the gas by the generalized second virial coefficient correlation. The equations and parameters you may need are listed as below.
\begin{parts}
\part [4] Please calculate the pressure by the ideal gas equation.
\part [18] Proceed {\bf{THREE}} iterations to get the answer for the pressure with the initial guess of $5\ bar$. $[\mbox{\bf{Note}}]$ You {\bf{MUST}} list the answer of each iteration.
\end{parts}
Where $Z$ is the compressibility factor; $B$ is the second virial coefficient of the virial equation of state; $P_r$, and $T_r$, are the reduced pressure and temperature, respectively. $\omega$ is the acentric factor; $T_c$ and $P_c$ are the temperature and pressure at the critical point, respectively; $B^0$, $B^1$, $Z^0$, $Z^1$ and $\hat{B}$ are the coefficients for this correlations.
\begin{align*}
& Z = 1 + \frac{BP}{RT} = 1 + \hat{B} \cdot \frac{P_r}{T_r}\quad\quad\quad\quad\quad\quad \hat{B} = \frac{B P_c}{R T_c} = B^0 + \omega B^1\quad\quad\quad\quad\; B^0 = 0.083 – \frac{0.422}{T^{1.6}_r}\\
& Z = 1 + B^0 \frac{P_r}{T_r} + \omega B^1 \frac{P_r}{T_r}\quad\quad\quad Z^0 = 1 + B^0 \frac{P_r}{T_r}\quad\quad\quad\ Z^1 = B^1 \frac{P_r}{T_r}\quad\quad\quad B^1 = 0.139 – \frac{0.172}{T^{4.2}_r}\\
& \omega = 0.200\quad\quad\quad\;\; T_c = 425.1\ K\quad\quad\quad\;\;\ P_c = 37.96\ bar
\end{align*}