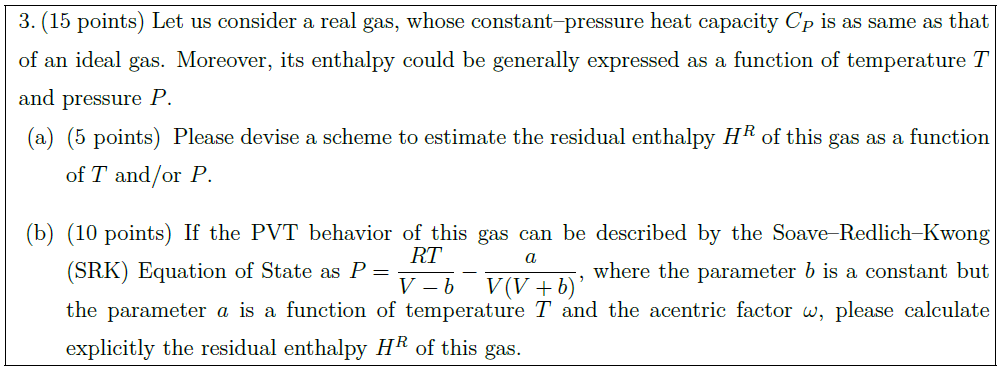
Solution:
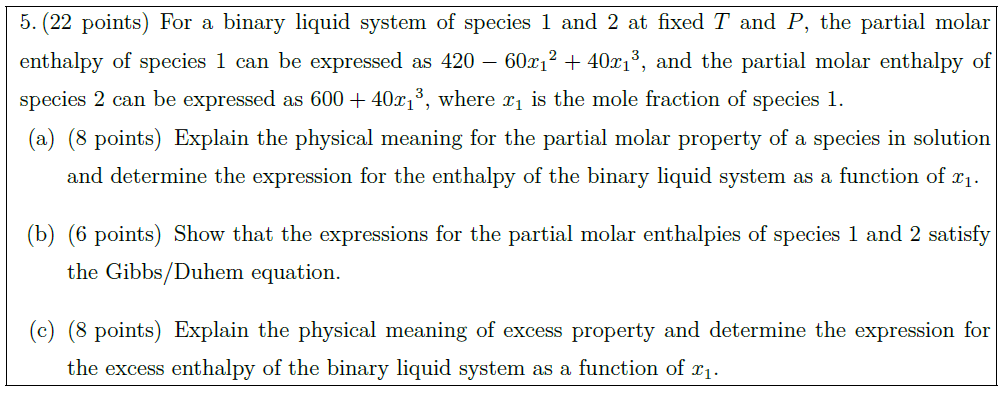
For a binary liquid system of species 1 and 2 at fixed $T$ and $P$, the partial molar enthalpy of species 1 can be expressed as $420 – 60 {x_1}^2 + 40 {x_1}^3$, and the partial molar enthalpy of species 2 can be expressed as $600 + 40 {x_1}^3$, where $x_1$ is the mole fraction of species 1.
\begin{parts}
\part [8] Explain the physical meaning for the partial molar property of a species in solution and determine the expression for the enthalpy of the binary liquid system as a function of $x_1$.
\part [6] Show that the expressions for the partial molar enthalpies of species 1 and 2 satisfy the Gibbs/Duhem equation.
\part [8] Explain the physical meaning of excess property and determine the expression for the excess enthalpy of the binary liquid system as a function of $x_1$.
\end{parts}