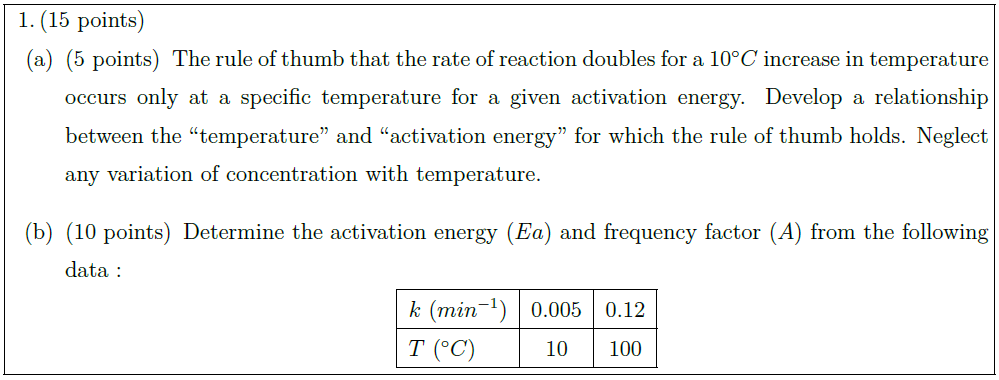
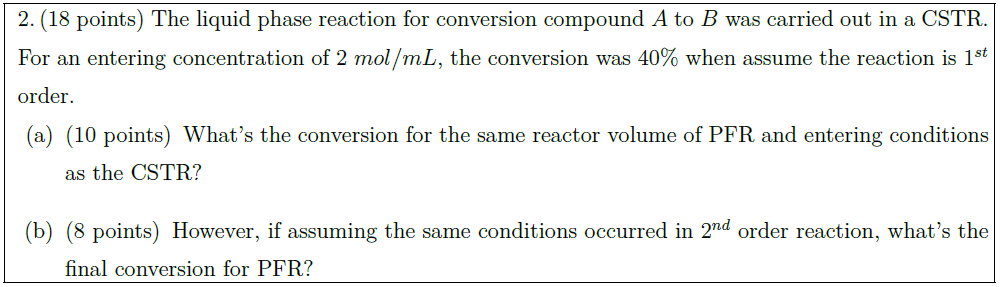
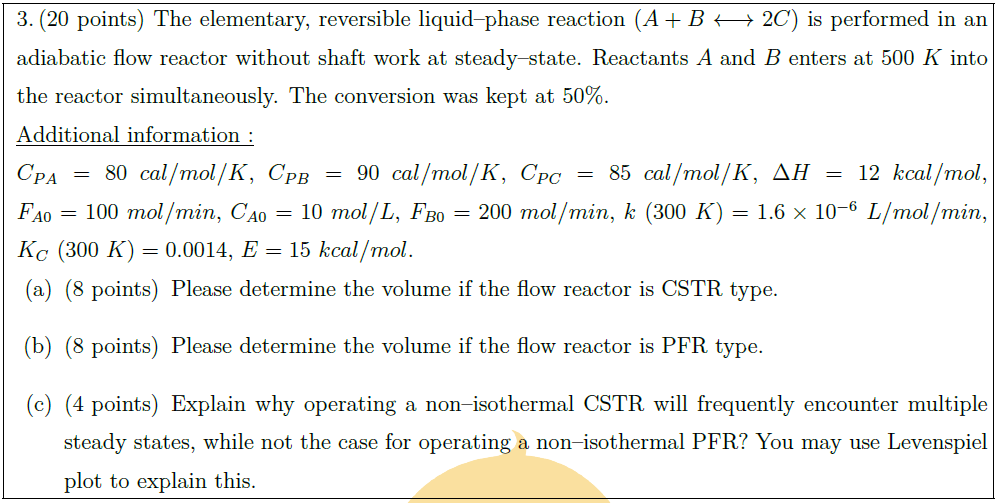
Solution:
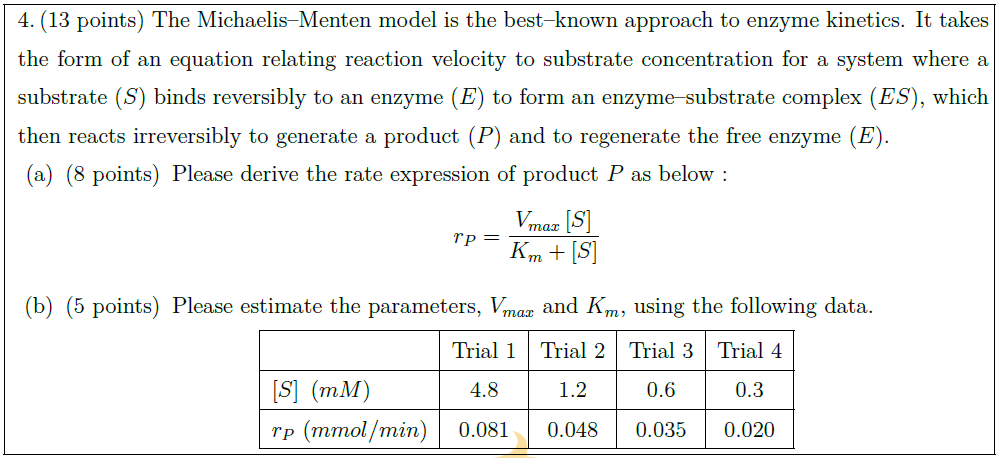
The Michaelis–Menten model is the best–known approach to enzyme kinetics. It takes the form of an equation relating reaction velocity to substrate concentration for a system where a substrate ($S$) binds reversibly to an enzyme ($E$) to form an enzyme–substrate complex ($ES$), which then reacts irreversibly to generate a product ($P$) and to regenerate the free enzyme ($E$).
\begin{parts}
\part [8] Please derive the rate expression of product $P$ as below :
\begin{align*}
r_P = \frac{V_{max} \left[ S \right]}{K_m + \left[ S \right]}
\end{align*}
\part [5] Please estimate the parameters, $V_{max}$ and $K_m$, using the following data.
\begin{center}
\begin{tabular}{|l|c|c|c|c|}
\hline
& Trial 1 & Trial 2 & Trial 3 & Trial 4 \\
\hline
$\left[ S \right]\ (mM)$ & 4.8 & 1.2 & 0.6 & 0.3 \\
\hline
$r_P\ (mmol/min)$ & 0.081 & 0.048 & 0.035 & 0.020 \\
\hline
\end{tabular}
\end{center}
\end{parts}