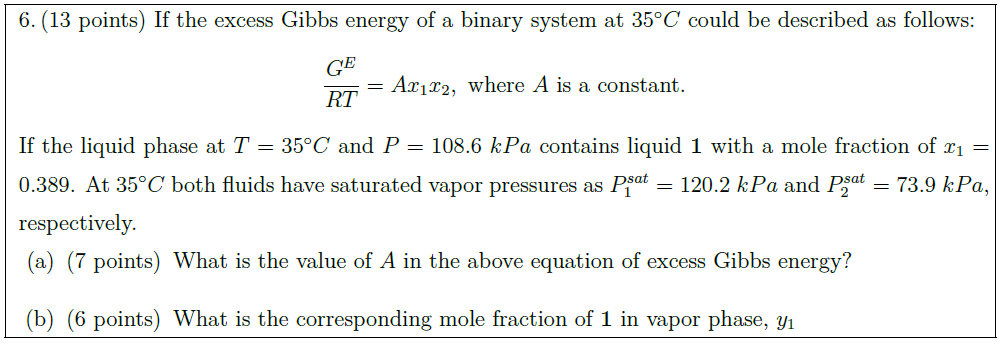
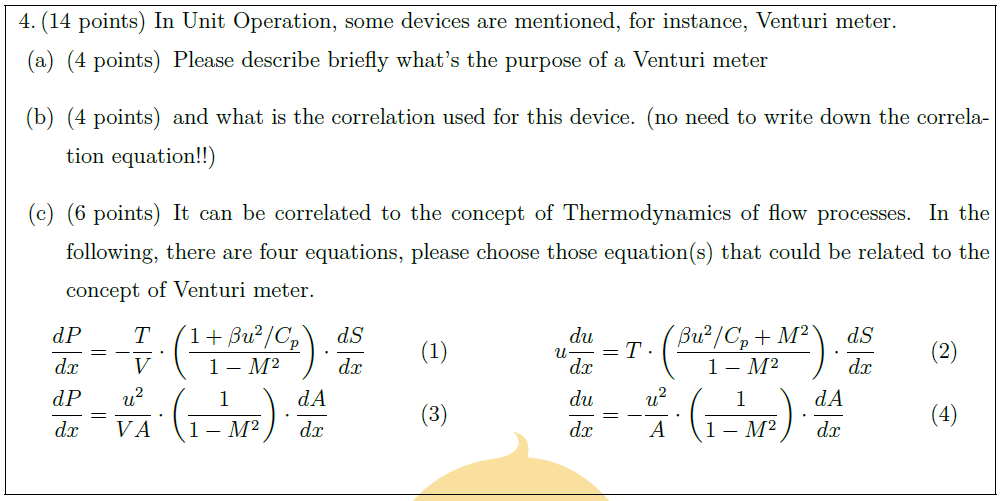
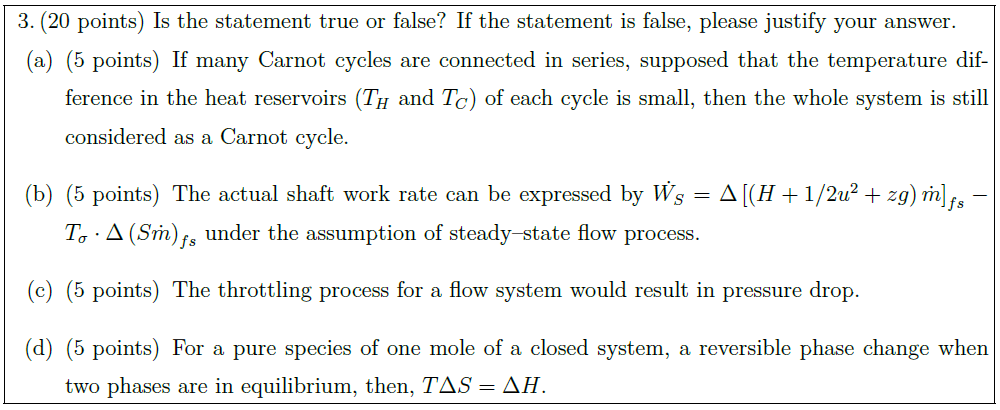
Solution:
Consider a liquid mixture made of $x_1$ moles of liquid {\bf 1} and $x_2$ moles of liquid {\bf 2} at a given temperature and pressure, in which $x_1 + x_2 = 1$. The van der Waals equations of state apply to both pure fluids ({\bf 1} and {\bf 2}) and the binary mixture, as follows:
\begin{align*}
P = \frac{R T}{V – b} – \frac{a}{V^2},\ \mbox{where $a$ and $b$ are two constants.}
\end{align*}
Theses two constants ($a$, $b$) for fluid {\bf 1}, and fluid {\bf 2} and liquid mixture are given as ($a_1$, $b_1$), ($a_2$, $b_2$), and ($a_m$, $b_m$), respectively. Moreover, the following relation holds:
\begin{align*}
b_m = x_1 \cdot b_1 + x_2 \cdot b_2 \quad \mbox{and} \quad \sqrt{a_m} = x_1 \cdot \sqrt{a_1} + x_2 \cdot \sqrt{a_2}.
\end{align*}
It is reasonable to expect the excess entropy $S^E$ near zero ($S^E = 0$) in this liquid mixture. What is the excess Gibbs energy of $G^E$ this liquid mixture? Please give $G^E$ in terms of $a_i$, $b_i$, and $x_i$ ($i = 1$ and $2$).