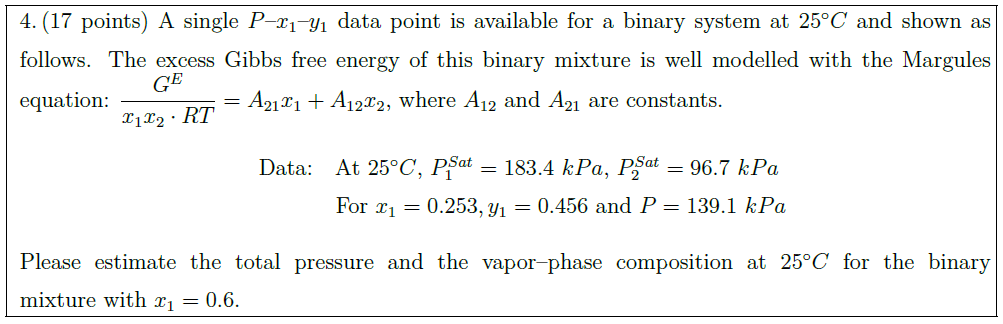
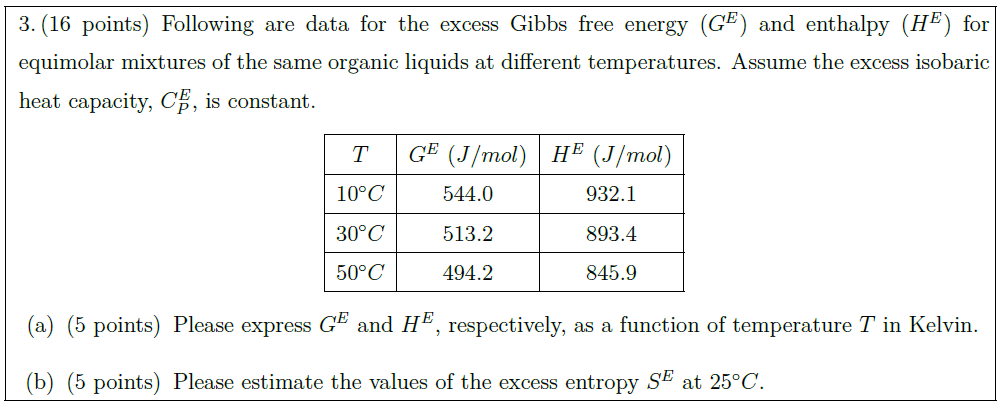
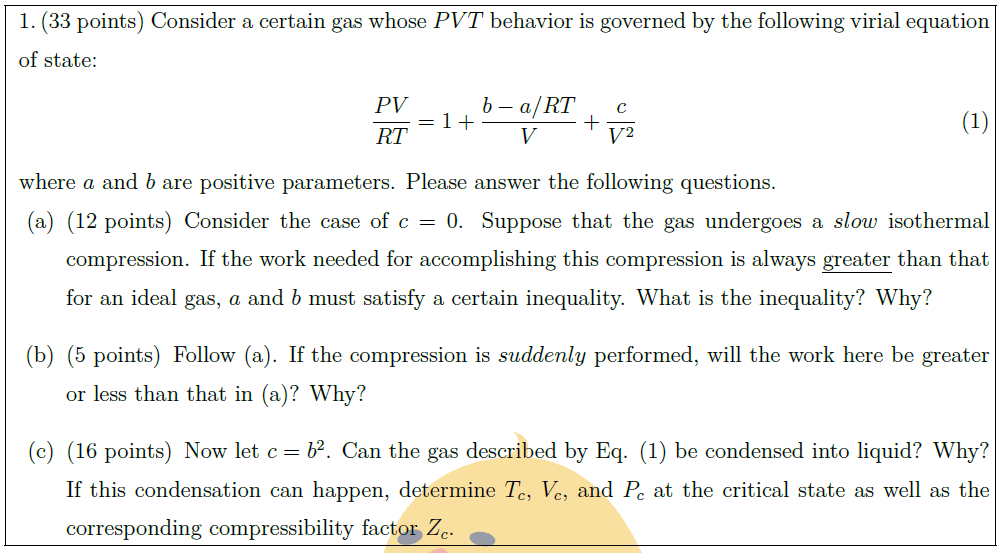
Solution:
\begin{parts}
\part [4] What is the main condition for the equation to establish?
\begin{align*}
\frac{S^R}{R} = – T \int_0^P \left( \frac{\partial Z}{\partial T} \right)_P \frac{dP}{P} – \int_0^P \left( Z – 1 \right) \frac{dP}{P}
\end{align*}
\part [4] Please draw a heat pump which includes constant heat reservoirs of $T_H$ (high temp) and $T_C$ (low temp), work ($W$), and heat ($Q_H$ and $Q_C$). \textbf{[Note]} You MUST indicate the directions by arrows.
\part [4] Follow (b), therefore, what is the definition of ”coefficient of performance” of a Carnot pump by Carnot’s theorem? (You are only allowed to use those symbols in (b)).
\part [4] Please write down the ”Carnot efficiency” of a Carnot engine. (Carnot’s theorem is applied again. Again, you are only allowed to use those symbols in (b)).
\part [4] Please explain the statement: ”For most gases at moderate $T$ and $P$, a reduction in $P$ at constant $H$ cause a decrease in $T$.
\part [14] Please draw the four steps of a Carnot cycle on a $P$ v.s $V$ diagram. \textbf{[Note]} The shape of the route and the different inclination (斜度) of the curves must be distinguishable (可區分). Please briefly describe each step. \textbf{[Note]} You MUST indicate the sequence by arrows. Sequence of the path is important.
\end{parts}