Solution:
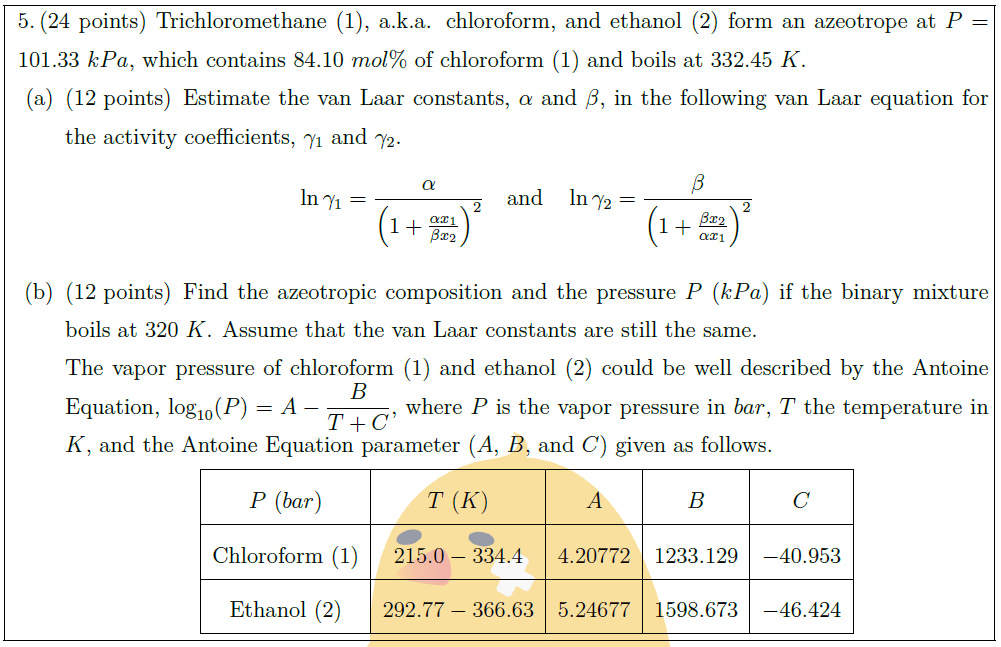
Trichloromethane (1), a.k.a. chloroform, and ethanol (2) form an azeotrope at $P = 101.33\ kPa$, which contains $84.10\ mol\%$ of chloroform (1) and boils at $332.45\ K$.
\begin{parts}
\part [12] Estimate the van Laar constants, $\alpha$ and $\beta$, in the following van Laar equation for the activity coefficients, $\gamma_1$ and $\gamma_2$.
\begin{align*}
\ln \gamma_1 = \frac{\alpha}{\left( 1 + \frac{\alpha x_1}{\beta x_2} \right)^2}\quad \mbox{and}\quad \ln \gamma_2 = \frac{\beta}{\left( 1 + \frac{\beta x_2}{\alpha x_1} \right)^2}
\end{align*}
\part [12] Find the azeotropic composition and the pressure $P$ ($kPa$) if the binary mixture boils at $320\ K$. Assume that the van Laar constants are still the same.\\
The vapor pressure of chloroform (1) and ethanol (2) could be well described by the Antoine Equation, $\displaystyle \log_{10} (P) = A – \frac{B}{T + C}$, where $P$ is the vapor pressure in $bar$, $T$ the temperature in $K$, and the Antoine Equation parameter ($A$, $B$, and $C$) given as follows.
\begin{center}
\begin{tabular}{|c|c|c|c|c|}
\hline
$P$ ($bar$) & $T$ ($K$) & $A$ & $B$ & $C$\\
\hline
Chloroform (1) & $215.0 – 334.4$ & $4.20772$ & $1233.129$ & $-40.953$\\
\hline
Ethanol (2) & $292.77 – 366.63$ & $5.24677$ & $1598.673$ & $-46.424$\\
\hline
\end{tabular}
\end{center}
\end{parts}