Solution:
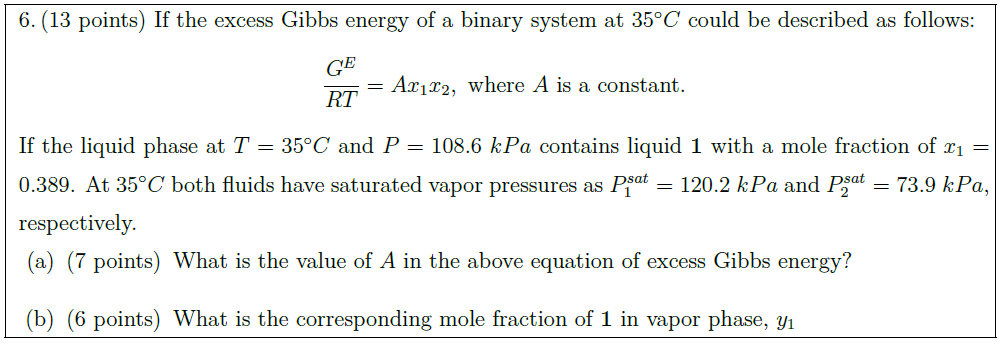
If the excess Gibbs energy of a binary system at $35^\circ C$ could be described as follows:
\begin{align*}
\frac{G^E}{R T} = A x_1 x_2,\ \mbox{where $A$ is a constant.}
\end{align*}
If the liquid phase at $T = 35^\circ C$ and $P = 108.6\ kPa$ contains liquid {\bf 1} with a mole fraction of $x_1 = 0.389$. At $35^\circ C$ both fluids have saturated vapor pressures as $P^{sat}_1 = 120.2\ kPa$ and $P^{sat}_2 = 73.9\ kPa$, respectively.
\begin{parts}
\part [7] What is the value of $A$ in the above equation of excess Gibbs energy?
\part [6] What is the corresponding mole fraction of {\bf 1} in vapor phase, $y_1$
\end{parts}