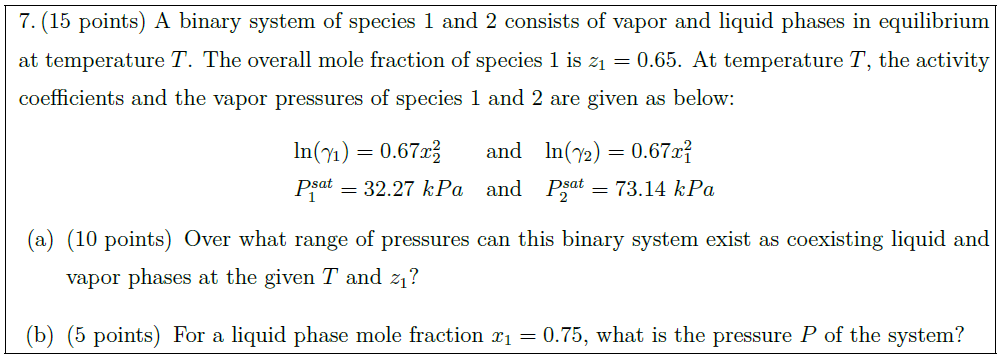
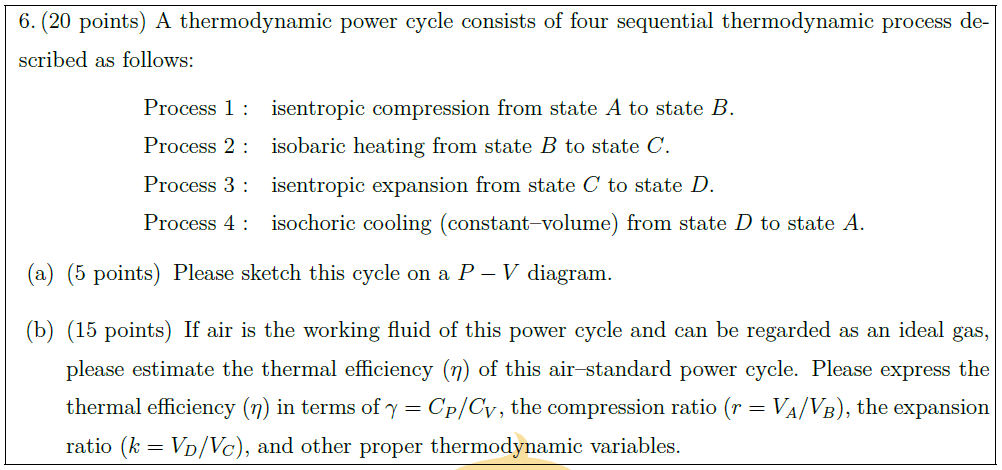
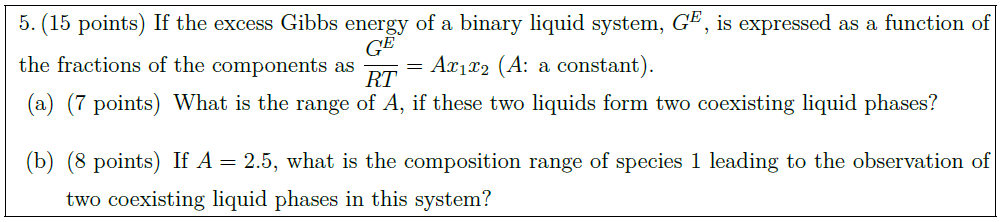
Solution:
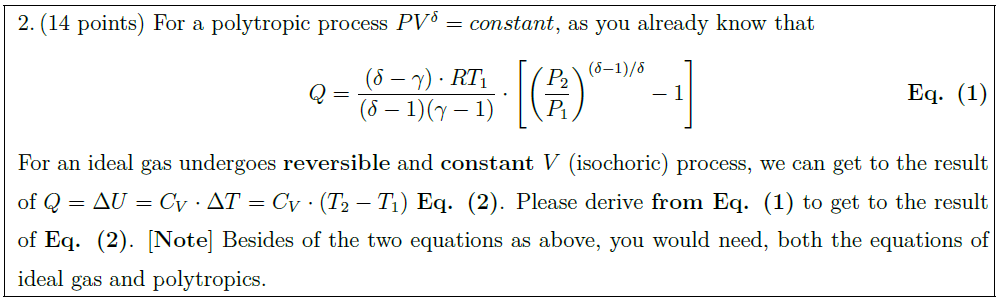
For a polytropic process $PV^\delta = constant$, as you already know that
\begin{align*}
Q = \frac{(\delta – \gamma) \cdot R T_1}{(\delta – 1) (\gamma – 1)} \cdot \left[ \left( \frac{P_2}{P_1} \right)^{\left( \delta – 1 \right) / \delta} – 1 \right] \tag*{{\bf Eq. (1)}}
\end{align*}
For an ideal gas undergoes {\bf reversible} and {\bf constant $V$} (isochoric) process, we can get to the result of $Q = \Delta U = C_V \cdot \Delta T = C_V \cdot (T_2 – T_1)$ {\bf Eq. (2)}. Please derive {\bf from Eq. (1)} to get to the result of {\bf Eq. (2)}. [{\bf Note}] Besides of the two equations as above, you would need, both the equations of ideal gas and polytropics.