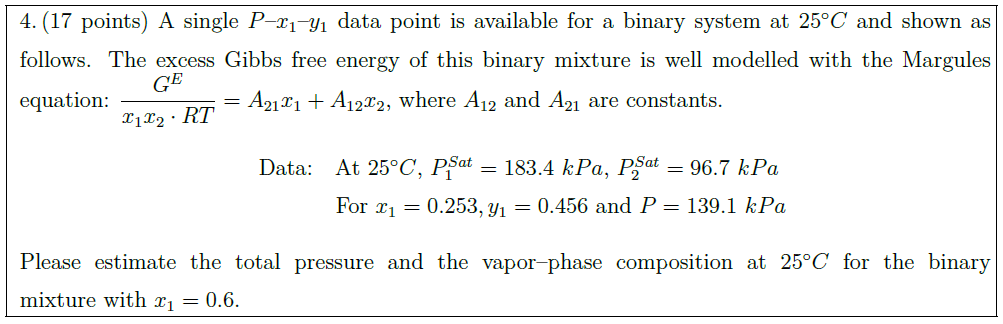
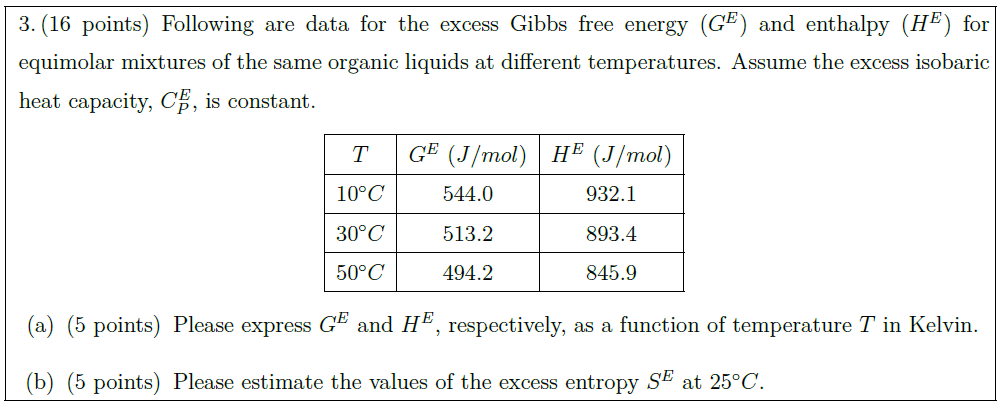
Solution:
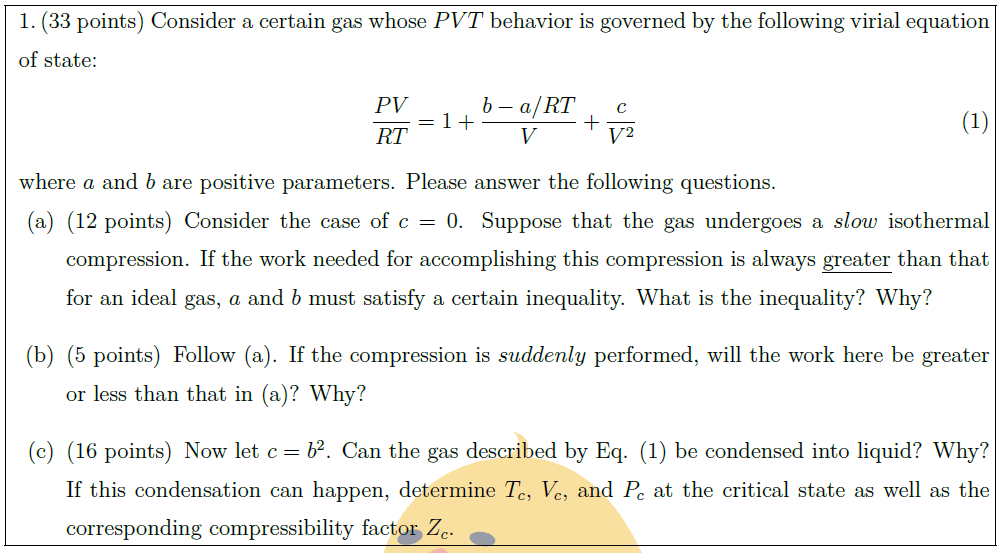
Consider a certain gas whose $PVT$ behavior is governed by the following virial equation of state:
\begin{align*}
\frac{PV}{RT} = 1 + \frac{b – a / RT}{V} + \frac{c}{V^2} \tag{1}
\end{align*}
where $a$ and $b$ are positive parameters. Please answer the following questions.
\begin{parts}
\part [12] Consider the case of $c = 0$. Suppose that the gas undergoes a $slow$ isothermal compression. If the work needed for accomplishing this compression is always \uline{greater} than that for an ideal gas, $a$ and $b$ must satisfy a certain inequality. What is the inequality? Why?
\part [5] Follow (a). If the compression is $suddenly$ performed, will the work here be greater or less than that in (a)? Why?
\part [16] Now let $c = b^2$. Can the gas described by Eq. (1) be condensed into liquid? Why? If this condensation can happen, determine $T_c$, $V_c$, and $P_c$ at the critical state as well as the corresponding compressibility factor $Z_c$.
\end{parts}