Solution:
An enzyme Glucose $6$–phosphatase has a $K_M$ value of $10^{-4}\ M$ and a $k_3$ value of $10^{4}\ min^{-1}$ at $37^\circ C$. The catalyzed reaction is the following :
\begin{align*}
\alpha \mbox{–} D \mbox{–Glucose }6 \mbox{–phosphate} \xrightarrow{\mbox{\scriptsize Glucose }\scriptstyle 6 \mbox{\scriptsize –phosphatase}} D \mbox{–Glucose}
\end{align*}
Which can be represented as
\begin{align*}
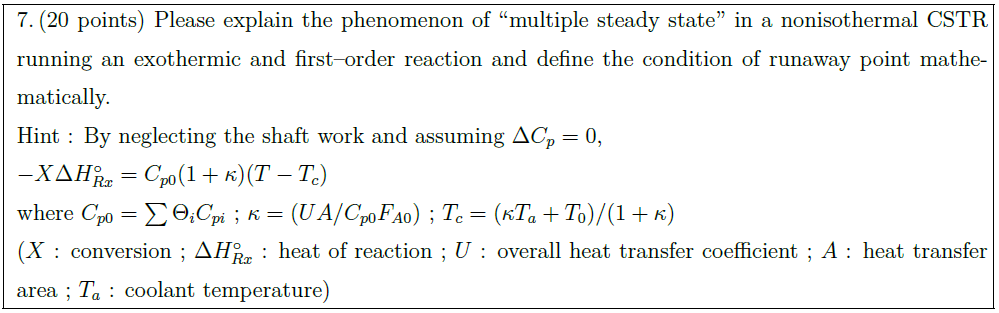
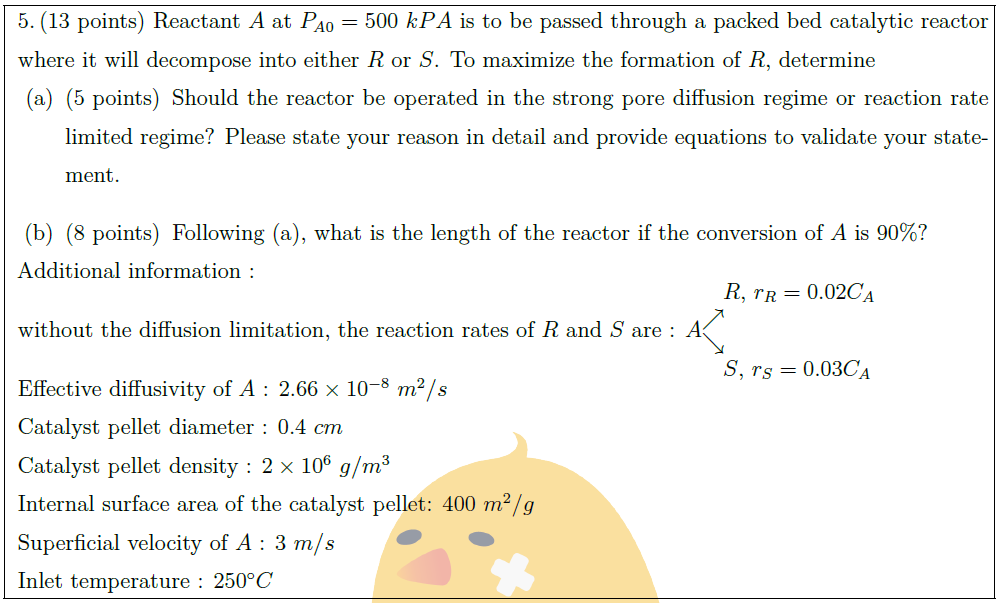
E + S \underset{k_2}{\overset{k_1}{\rightleftharpoons}} ES \xrightarrow{k_3} E + P
\end{align*}
Where $S$ is $\alpha$–D–Glucose $6$–phosphate and $P$ is D–Glucose, $E$ is the enzyme Glucose $6$–phosphatase. The enzyme is not stable at $37^\circ C$ and the amount of active enzyme will decrease along time :
\begin{align*}
E_{act} = E_0 \mbox{{\textit{\large e}}}^{- k_d t}
\end{align*}
Where $E_0$ is the initial enzyme concentration and $k_d = 0.1\ min^{-1}$. In an experiment, a certain amount of enzyme and $0.02\ M$ of $\alpha$–D–Glucose $6$–phosphate is added into a batch reactor and incubated at $37^\circ C$. After $12$ hours, the concentration of D–Glucose is fond to be $0.002\ M$. What is the initial concentration of enzyme ($E_0$)?